Question

Levi wants to order the fractions 1/3, 2/5, and 11/30 in descending order? How can you help him by using a common denominator? Explain and order the fractions in descending order.

Answer 1

The correct order of fractions in descending order will be:

`(2)/(5), (11)/(30), (1)/(3)`

Explanation:

Given fraction:

`(1)/(3), (2)/(5), (11)/(30)`

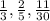
To arrange them in descending order.

Solution:

In order to arrange the fractions in descending order, we will have to find the least common denominators.

To find the least common denominator, we will find the least common multiple of the denominators 3,5, and 30.

Since 30 is a common multiple of all 3 numbers, so it will be the least common denominator.

So, we multiply the numerators and denominators with same numbers in order to make the denominators = 30.

So, we have:

`(1)/(3), (2)/(5), (11)/(30)`

⇒
`(1* 10)/(3* 10), (2* 6)/(5* 6), (11* 1)/(30* 1)`

⇒
`(10)/(30), (12)/(30), (11)/(30)`

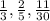
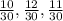
Now, we compare the numerators and arrange them accordingly.

`(12)/(30) > (11)/(30) > (10)/(30)`

So, the correct order of fractions in descending order will be:

`(2)/(5), (11)/(30), (1)/(3)`