Answer:
Step-by-step explanation:
Gravitational Potential Energy at earth surface
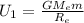
Gravitational Potential Energy at height h is
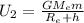
Energy required to lift the satellite
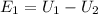
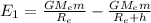
Now Energy required to orbit around the earth

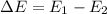
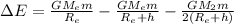
 (given)
(given)
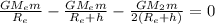
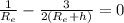
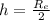
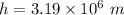
(b)For greater height
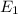 is greater than
is greater than

thus energy to lift the satellite is more than orbiting around earth