Question

Which expression is equivalent to StartRoot StartFraction 25 x Superscript 9 Baseline y Superscript 3 Baseline Over 64 x Superscript 6 Baseline y Superscript 11 Baseline EndFraction EndRoot? Assume x Greater-than 0 and y > 0.

Answer 1

D On edge.

Explanation:

Its Very Simple i Took it just now :D

Answer 2

`\displaystyle (5x)/(8y^(4))√(x)`

Explanation:

Simplfy in Algebra

We have the following expression

`\displaystyle E=\sqrt{(25x^9y^3)/(64x^6y^(11))}`

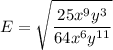
Simplifying like factors in the denominator and numerator

`\displaystyle E=\sqrt{(25x^3)/(64y^(8))}`

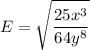
All the factors are perfect squares except
`x^3`, thus we rewrite:

`\displaystyle E=\sqrt{(25xx^2)/(64y^(8))}`

Taking the square root of all the perfect square factors:

`\boxed{\displaystyle E=(5x)/(8y^(4))√(x)}`