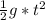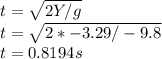Question

A daring ranch hand sitting on a tree limb wishes to drop vertically onto a horse galloping under the tree. The constant speed of the horse is 14.5 m/s, and the distance from the limb to the level of the saddle is 3.29 m.

(a) What must be the horizontal distance between the saddle and limb when the ranch hand makes his move?
(b) How long is he in the air?

Answer 1

For Part A:

ΔX=11.8813 m

Part B:

`t=0.8194 s`

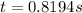
Step-by-step explanation:

Note: In order to find Part A we first have to find Part B i.e time

Data given:

`V_i=0`

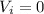
a=-9.8m/s^2 (-ve is because ranch is falling down)

Δy=-3.29m (-ve is because ranch is falling down)

Second equation of Motion:

Δy=
`V_i*t+(1)/(2)g*t^2`

V_i=0, Equation will become

Δy=
`(1)/(2)g*t^2`

`t=√(2Y/g) \\t=√(2*-3.29/-9.8) \\t=0.8194 s`

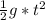
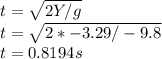
For Part A:

Again:

Second equation of Motion:

ΔX=
`V_i*t+(1)/(2)a*t^2`

Since velocity is constant a=0

V_i=14.5m/s, t=0.8194 sec

ΔX=
`V_i*t`

ΔX=
`14.5*0.8194`

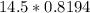
ΔX=11.8813 m

Part B: (Calculated above)

Data given:

`V_i=0`

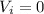
a=-9.8m/s^2 (-ve is because ranch is falling down)

Δy=-3.29m (-ve is because ranch is falling down)

Second equation of Motion:

Δy=
`V_i*t+(1)/(2)g*t^2`

V_i=0, Equation will become

Δy=
`(1)/(2)g*t^2`

`t=√(2Y/g) \\t=√(2*-3.29/-9.8) \\t=0.8194 s`