Answer:
The option
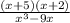 is correct
is correct
The difference of the given expression is

Explanation:
Given expression is

To find the difference of the given expression as below :

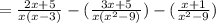
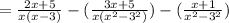
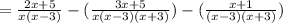
( using the formula
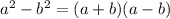 )
)
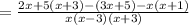
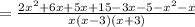 (adding the like terms)
(adding the like terms)
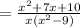 ( by factoring the quadratic polynomial )
( by factoring the quadratic polynomial )
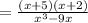
Therefore

Therefore the difference of the given expression is
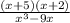
Therefore option
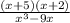 is correct
is correct