Question

A ball of mass 0.165 kg is dropped from rest from a height of 1.25 m. It rebounds from the floor to reach a height of 0.940 m. What impulse was given to the ball by the floor?

Answer 1

I = 1.525 kg.m/s

Step-by-step explanation:

given,

mass of the ball = 0.165 Kg

height of drop, h = 1.25 m

ball rebound and reach to height, h' = 0.940 m

impulse = ?

using conservation of energy

Potential energy is converted into kinetic energy

`mgh = (1)/(2)mv^2`

`v=√(2gh)`

`v=√(2* 9.8 * 1.25)`

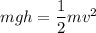
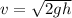
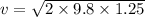
v = 4.95 m/s

velocity of the ball after rebound

again using conservation of energy

`mgh = (1)/(2)mv'^2`

`v'=√(2gh)`

`v'=√(2* 9.8 * 0.94)`

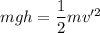
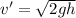
v' = 4.29 m/s

impulse is equal to change in momentum

I = m ( v' - v )

I = 0.165 x ( 4.29 - (-4.95))

I = 1.525 kg.m/s