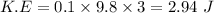Question

A ball of mass 100g falls from a height of 3m on to a horizontal surface and rebounds to a height of 2m. calculate the change in momentum and the kinetic energy of the ball it strikes the surface​

Answer 1

The change in momentum is equal to 1.3928 kg.m/s².

The kinetic energy of the ball as it strikes the surface = 2.94 Joules

Step-by-step explanation:

Given:

Mass of the ball = 100 g = 100 × 10⁻³ kg = m = 0.1 kg

Height from which ball falls = h = 3 m

Height to which the ball rebounds = h' = 2 m

g= acceleration due to gravity = g = 9.8 m/s²

1. A ball dropped from a height of 3 m, hits the horizontal surface and bounces back. This will cause a change in momentum.

The velocity of the ball before hitting the surface and after hitting the surface is different; causing a change in momentum (Δp).

Since momentum (p) = mass (m) × velocity (v) :
`p=m* v`

Δp =
`m* V_\ after`
`-\ m* V_\ before` =
`m* (V_\ after`
`\ -\ V_\ before`
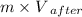
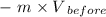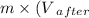
`)` ...........................(1)

Velocity (v) is given by
`v = √(2gh)`

`V_\ before` =
`√(2* g* h) = √(2* 9.8* 3) = √(58.8) = 7.668\ m/s^2`

`V_\ after` =
`√(2* g* h') = √(2* 9.8* 2) = √(39.2) = 6.2609\ m/s^2`

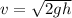
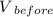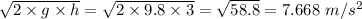
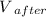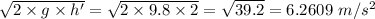
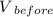`V_\ before` is a decreasing quantity (Ball dropped from 3 m) until it becomes equal to
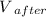`V_\ after` and hence is a negative vector (negative in sign and opposite in direction to
`V_\ after`.)

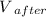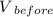`V_\ before` = (-7.668 m/s²)

From (1), for m = 0.1 kg, Δp becomes;

Δp =
`m* (V_\ after`
`\ -\ V_\ before`
`)` =
`0.1( 6.2609 - ( - 7.668)) = 0.1* 13.9289 = 1.3928\ kg.m/s`

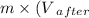
2. As a ball falls to the surface, its gravitational potential energy is transformed into kinetic energy. This kinetic energy keeps on increasing with increase in momentum until it strikes the surface.

K.E of ball just before it strikes the surface from 3 m height =
`K.E = mgh`

`K.E = 0.1* 9.8* 3 = 2.94\ J`