As the question is unable to be understood/incomplete hence let's discuss the complete question (most probable question) and the answer for that.
Updated Question:
"A bicycle manufacturing company makes a particular type of bike. Each child bike requires 4 hours to build and 4 hours to test. Each adult bike requires 6 hours to build and 4 hours to test. With the number of workers, the company is able to have up to 120 hours of building time and 100 hours of testing time for a week. If c represents child bikes and a represents adult bikes, determine which system of inequality best explains whether the company can build 20 child bikes and 6 adult bikes in the week. No, because the bike order does not meet the restrictions of 4c + 6a ≤ 120 and 4c + 4a ≤ 100 No, because the bike order does not meet the restrictions of 4c + 4a ≤ 120 and 6c + 4a ≤ 100 Yes, because the bike order meets the restrictions of 4c + 6a ≤ 120 and 4c + 4a ≤ 100 Yes, because the bike order meets the restrictions of 4c + 4a ≤ 120 and 6c + 4a ≤ 100"
Answer:
Correct answer for this question is first option because it satisfies the first inequality but not the second one.
Step by Step Explanation:
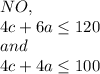
First Inequality:
4(20)+6(6)≤120
80+36≤120
116≤120 ⇒ TRUE
Second Inequality:
4(20)+4(6)≤100
80+24≤100
104≤100 ⇒ FALSE