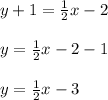Question

ABC has vertices at A(5,-1), B(9, -9), and C(4,-1). Write the equation of the line in slope-intercept form that is perpendicular to AB and passes through point C

Answer 1

`y=(1)/(2)x-3`

Explanation:

step 1

Find the slope of AB

we know that

The formula to calculate the slope between two points is equal to

`m=(y2-y1)/(x2-x1)`

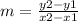
we have

A(5,-1), B(9, -9)

substitute the values

`m=(-9+1)/(9-5)`

`m=(-8)/(4)`

`m=-2`

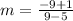
step 2

Find the slope of the line that is perpendicular to AB

we know that

If two lines are perpendicular, their their slopes are opposite reciprocal (the product of their slopes is equal to -1)

we have

`m_A_B=-2`

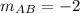
therefore

The slope of the perpendicular line is

`m=(1)/(2)`

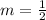
step 3

Find the equation of the line in point slope form

`y-y1=m(x-x1)`

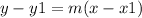
we have

`m=(1)/(2)`

`C(4,-1)`

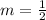
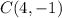
substitute

`y+1=(1)/(2)(x-4)`

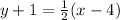
step 4

Convert to slope intercept form

Isolate the variable y

`y+1=(1)/(2)x-2\\\\y=(1)/(2)x-2-1\\\\y=(1)/(2)x-3`