Answer:
24.2% students received grades with z-scores between 0.15 and 0.85
Explanation:
We are given the following in the question:
The grades of a benchmark test for North High School were normally distributed.
WE have to find the percentage of students that received grades with z-scores between 0.15 and 0.85.
Formula:

P(score between 0.15 < z < 0.85)
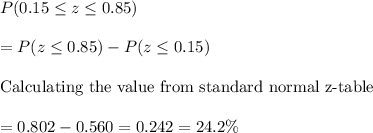
24.2% students received grades with z-scores between 0.15 and 0.85