Question

A round pipe of varying diameter carries petroleum from a wellhead to a refinery. At the wellhead, the pipe's diameter is 50.9 cm ( 0.509 m) and the flow speed of the petroleum is 11.5 m/s. At the refinery, the petroleum flows at 5.25 m/s. What is the volume flow rate of the petroleum along the pipe and what is the pipe's diameter at the refinery

Answer 1

Flow rate 2.34 m3/s

Diameter 0.754 m

Step-by-step explanation:

Assuming steady flow, the volume flow rate along the pipe will always be constant, and equals to the product of flow speed and cross-section area.

The area at the well head is

`A = \pi r_w^2 = \pi (0.509/2)^2 = 0.203 m^2`

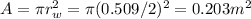
So the volume flow rate along the pipe is

`\dot{V} = Av = 0.203 * 11.5 = 2.34 m^3/s`

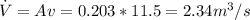
We can use the similar logic to find the cross-section area at the refinery

`A_r = \dot{V}/v_r = 2.34 / 5.25 = 0.446 m^2`

The radius of the pipe at the refinery is:

`A_r = \pi r^2`

`r^2 =A_r/\pi = 0.446/\pi = 0.141`

`r = √(0.141) = 0.377m`

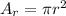
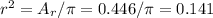
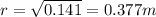
So the diameter is twice the radius = 0.38*2 = 0.754m