Question

In A RST, RT = 4, ST = 8, and TX bisects < RTS.

Which of the following proportions must be true?

Answer 1

RX over RT equals SX over ST

Explanation:

Answer 2

`(RX)/(RT)=(SX)/(ST)`

Explanation:

The options of the questions are

RX/ST = TR/XS

TX/XR = ST/RT

RX/RT = SX/ST

RX/RS = XS/ST

we know that

The Angle-Bisector theorem states that if a ray bisects an angle of a triangle, then it divides the opposite side into segments that are proportional to the other two sides

In this problem we have

TX bisect < RTS

then

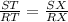`(ST)/(RT)=(SX)/(RX)` ----> by Angle Bisector Theorem

Rewrite the expression

`(RX)/(RT)=(SX)/(ST)`