Question

Review. As an astronaut, you observe a small planet to be spherical. After landing on the planet, you set off, walking always straight ahead, and find yourself returning to your spacecraft from the opposite side after completing a lap of 25.0 km. You hold a hammer and a falcon feather at a height of 1.40 m, release them, and observe that they fall together to the surface in 29.2 s. Determine the mass of the planet

Answer 1

To find the mass of the planet we will apply the relationship of the given circumference of the planet with the given data and thus find the radius of the planet. From the kinematic equations of motion we will find the gravitational acceleration of the planet, and under the description of this value by Newton's laws the mass of the planet, that is,

The circumference of the planet is,

`\phi = 25.1m`

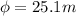
Under the mathematical value the radius would be

`\phi = 2\pi r`

`r = (25)/(2\pi)`

`r = 3.9788km`

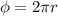
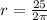
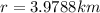
Using second equation of motion

`x = (1)/(2) at^2`

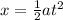
Replacing the values given,

`1.4 = (1)/(2) a (29.2)^2`

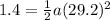
Rearranging and solving for 'a' we have,

`a = 0.003283m/s^2`

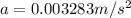
Using the value of acceleration due to gravity from Newton's law we have that

`a = (GM)/(r^2)`

Here,

r = Radius of the planet

G = Gravitational Universal constant

M = Mass of the Planet

`((6.67*10^(-11))*M)/((3.9788*10^3)^2) = 0.003283`

`M = 7.79201*10^(14)kg`

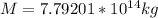
Therefore the mass of this planet is
`7.79201*10^(14)kg`