Question

A raft of mass 199 kg carries two swimmers of mass 52 kg and 70 kg. The raft is initially floating at rest. The two swimmers simultaneously dive off opposite ends of the raft, each with a horizontal velocity of 4 m/s. With what velocity the raft start to move

Answer 1

To solve this problem we will apply the concept related to the conservation of the Momentum. We will then start considering that the amount of initial momentum must be equal to the amount of final momentum. Considering that all the objects at the initial moment have the same initial velocity (Zero, since they start from rest) the final moment will be equivalent to the multiplication of the mass of each object by the velocity of each object, so

Initial Momentum = Final Momentum

`(m_B+m_1+m_2)v_i = m_1v_1+m_2v_2+m_Bv_B`

Here,

`m_B` = mass of Raft

`m_1` = Mass of swimmers 1

`m_2` = Mass of swimmers 2

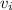`v_i` = Initial velocity (of the three objects)

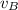`v_B` = Velocity of Raft

Replacing,

`(199+52+70)*0 = (52)(4)+(70)(-4)+199v_B`

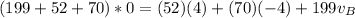
Solving for
`v_B`

`vB = (72)/(199)`

`v_B = 0.3618m/s`

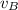
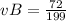
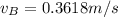
Therefore the velocity the rarft start to move is 0.3618m/s