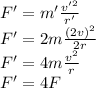Answer:
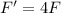
Step-by-step explanation:
According to Newton's second law, the tension in the string is equal to the centripetal force, since the mass is under an uniform circular motion:
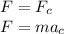
Here
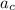 is the centripetal acceleration, which is defined as:
is the centripetal acceleration, which is defined as:
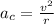
So, replacing:
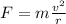
In this case we have
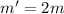 ,
,
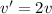 and
and
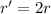 . Thus, the tension required to mantain uniform circular motion is:
. Thus, the tension required to mantain uniform circular motion is: