Question

Newly discovered planet has twice the mass and three times the radius of the earth. What is the free-fall acceleration at its surface, in terms of the free-fall acceleration g at the surface of the earth?

Answer 1

2 g/9

Step-by-step explanation:

mass of planet, Mp = 2 x Me

radius of planet, Rp = 3 x Re

Where, Me is the mass of earth and Re is the radius of earth.

The formula for acceleration due to gravity on earth is given by

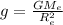`g = (GM_(e))/(R_(e)^(2))` .... (1)

The acceleration due to gravity on the planet is given by

`g' = (GM_(p))/(R_(p)^(2))`

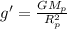
By substituting the values, we get

`g' = (2GM_(e))/(9R_(e)^(2))` ..... (2)

Divide equation (2) by equation (1), we get

g'/g = 2/9

g' = 2 g/9

Thus, the acceleration due to gravity on th enew planet is 2 g/9.

Answer 2

`g_n=(2)/(9)g`

Step-by-step explanation:

M = Mass of Earth

G = Gravitational constant

R = Radius of Earth

The acceleration due to gravity on Earth is

`g=(GM)/(R^2)`

On new planet

`g_n=(G2M)/((3R)^2)\\\Rightarrow g_n=(2GM)/(9R^2)`

Dividing the two equations we get

`(g_n)/(g)=((2GM)/(9R^2))/((GM)/(R^2))\\\Rightarrow (g_n)/(g)=(2)/(9)\\\Rightarrow g_n=(2)/(9)g`

The acceleration due to gravity on the other planet is
`g_n=(2)/(9)g`