390 gallons of 90 % antifreeze must be mixed with 60 gallons of 15% antifreeze to get a mixture that is 80%
Solution:
Let "x" be the gallons of 90 % antifreeze
60 gallons of 15 % antifreeze
Then, (x + 60) is the gallons present in final mixture that is 80 %
Therefore, x gallons of 90 % antifreeze is mixed with 60 gallons of 15 % antifreeze to get a mixture that is (x + 60) gallons of 80 %
Thus, a equation is framed:
90 % of x + 15 % of 60 = 80 % of (x + 60)
Solve the above equation for "x"
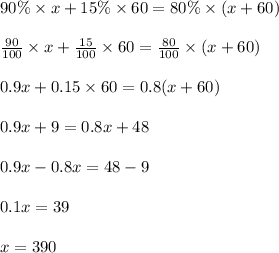
Thus 390 gallons of 90 % antifreeze must be mixed