Answer:
1.65 kg.m/s
Step-by-step explanation:
mass of ball (m) = 0.13 kg
initial speed (u) = 18 m/s
acceleration due to gravity (g) = 9.81 m/s^{2}
angle of projection (p) = 90°
to find the momentum half way to its maximum height we first have to know the maximum height, and the velocity at the maximum height.
maximum height (h) =
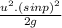
maximum height (h) =
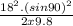 = 16.53 m
= 16.53 m
velocity at half the maximum height (v) can be gotten from the equation of motion
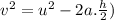 ( the negative sign is because the ball is moving upwards)
( the negative sign is because the ball is moving upwards)
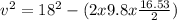

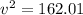
v =
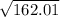
v= 12.72 m/s
momentum of the ball half way to its maximum height = mass x velocity = 0.13 x 12.72 = 1.65 kg.m/s