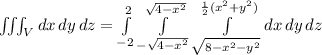Answer:
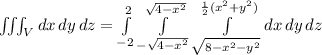
Explanation:
The common region enclosed above the paraboloid
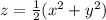 and below the sphere
and below the sphere
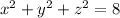 is the solid.
is the solid.
Let's find the limit bounds for
 .
.
The region is bounded above by the sphere
 .
.
Isolate
 from the equation above.
from the equation above.
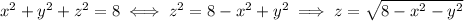
On the other hand, the region is bounded below by the paraboloid
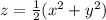
Therefore, we obtain
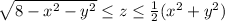
Now, we need to find the intersection of the sphere and the paraboloid. To do so, we need to solve the following system of equations
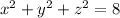
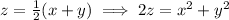 .
.
Substitute the second equation in the first equation. We obtain

Hence, the paraboloid and the sphere intersect when
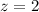 .
.
Substituting
 for
for
 in the equation above gives
in the equation above gives

which is a circle with a radius
 .
.
Now, we can find the bond for
 and
and
 .
.
For
 , we obtain
, we obtain
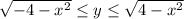 .
.
For
 , we have
, we have
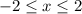 .
.
Therefore, the needed triple integral is