Question

2. A 16.0 kg canoe moving to the left at 12.0 m/s makes an elastic head-on collison with a 4.0 kg raft moving to the right at 6.0 m/s. After the collision, the raft moves to the left at 22.7 m/s. Disregard any effects of the water. (a) Find the velocity of the canoe after the collision. (b) Verify your anser by calculating the total kinetic energy before and after the collision.

Answer 1

(a) 4.83 m/s to the left

(b) 1224 J

Step-by-step explanation:

Using the law of conservation, the initial momentum equals the final momentum. Taking left as positive while right as negative then

momentum, p=mv where m is mass and v is velocity

After the collision, the canoe keep their same masses

`m_1v_1+m_2v_2=m_1v_3+m_2v_4`

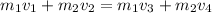
m and v represent mass and velocity respectively, the subscripts 1 and 2 are the initial velocities and masses while subscripts 3 and 4 are final velocities

Substituting
`m_1` for 16 Kg,
`m_2` for 4 Kg,
`v_1` for 12 m/s,
`v_2` for -6 m/s since it's towards right and we already mentioned that right is negative,
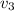`v_3` for 22.7 m/s then

`(16 Kg* 12 m/s)+(4 Kg* -6 m/s)=(16 Kg* v_4)+ (4 Kg* 22.7 m/s)`

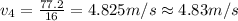`v_4=\frac {77.2}{16}=4.825 m/s\approx 4.83 m/s` hence direction is to the left

(b)

We know that kinetic energy is given by
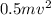`0.5mv^(2)` and by substituting the given values of mass and velocity, in this case not considering direction then we add the values of the two objects in question, and get the total before, and add the values of the two objects after and get the total after

Kinetic energy before collision=KE=
`0.5mv^(2)=0.5(16*12^(2)+4*6^(2))=1224 J`

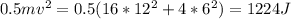
Kinetic energy after collision=KE=

`0.5mv^(2)=0.5(16 Kg* 4.83^(2)+ 4 Kg* 22.7^(2))=1217.211 J`

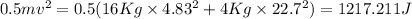
The two values of KE before and after collision are almost equal hence we conclude that energy is conserved.