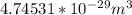Answer:
Volume of face centered cubic cell=
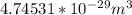
Step-by-step explanation:
Consider the face centered cubic cell:
1 atom at each corner of cube.
1 atom at center of each face.
Consider the one face (ABCD) as shown in attachment for calculation:
Length of the all sides of face centered cubic cell is L.
Volume of face centered cubic cell= L^3
Now Consider the figure shown in attachment:
According to Pythagoras theorem on ΔADC.
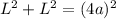 (a is the atomic radius)
(a is the atomic radius)
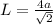 (Put in the formula of Volume)
(Put in the formula of Volume)
Volume of face centered cubic cell= L^3
Volume of face centered cubic cell=
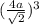
Volume of face centered cubic cell=
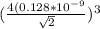
Volume of face centered cubic cell=