Answer:
○

Step-by-step Step-by-step explanation:
Use the Law of Sines to find the length of the second edge:
Solving for Angles
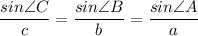
Use
 towards the end or you will throw your result off!
towards the end or you will throw your result off!
Solving for Edges
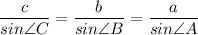
Let us get to wourk:

I am joyous to assist you at any time.