Question

In rectangle ABCD, point E lies half way between sides AB and CD and halfway between sides AD and BC. If AB=10 and BC=2, what is the area of the shaded region?

Answer 1

The area of the shaded region in the rectangle is 5 square units, calculated by multiplying the length and width of one of the four equal smaller rectangles created by midpoint E.

Step-by-step explanation:

To determine the area of the shaded region in rectangle ABCD with point E lying halfway between the sides, we recognize that point E creates four smaller rectangles of equal area within ABCD. Given that AB is 10 units and BC is 2 units, the dimensions of each smaller rectangle will be 5 units by 1 unit, since E is the midpoint of the sides.

The area of a rectangle is calculated by multiplying the length by the width. Therefore, the area of one smaller rectangle is 5 units × 1 unit = 5 square units. To find the area of the shaded region, which comprises one of these smaller rectangles, we use the same formula: 5 square units.

Thus, the area of the shaded region within rectangle ABCD is 5 square units.

Answer 2

Area of shaded region is
`10 \ units^2`.

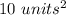Step-by-step explanation:

We are attaching the diagram for your reference.

Given:

AB = 10

BC = 2

We need to find the Area of the shaded region.

Area of the shaded region is equal to sum of Area of triangle ABE and Area of triangle CDE.

Now first we will find area of both the triangles.

Now we know that Area of triangle is half times base times height.

In Δ ABE,

base AB = 10 (given)

Since given point E lies half way between sides AB and CD and halfway between sides AD and BC.

So height h is,

height h =
`(BC)/(2) = (2)/(2) = 1`

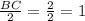
Area of Δ ABE =
`(1)/(2) * base * height = (1)/(2)* 10* 1 = 5\ units^2`

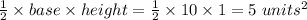
In Δ CDE,

base CD = AB = 10 (given it is a rectangle and opposite side of rectangle are equal)

Since given point E lies half way between sides AB and CD and halfway between sides AD and BC.

So height h is,

height h =
`(BC)/(2) = (2)/(2) = 1`

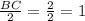
Area of Δ CDE =
`(1)/(2) * base * height = (1)/(2)* 10* 1 = 5\ units^2`

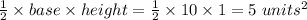
Now Area of shaded region = Area of Δ ABE + Area of Δ CDE =
`5\ units^2+ 5\ units^2 = 10\ units^2`

Hence Area of shaded region is
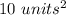`10 \ units^2`.