Answer:
The net charge on the object is 0.1328 μC.
Step-by-step explanation:
Given that,
Proton
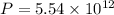
Electron
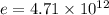
We need to calculate the net charge on the object
Using formula of net charge
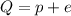
Where, Q = net charge
p = protons
e = electron
Put the value into the formula
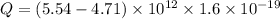
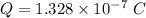
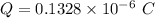
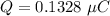
Hence, The net charge on the object is 0.1328 μC.