Question

F(x) = 5x^5 + 10x^4 + 3x^3 + 8x^2 - 6x - 3; c = 3

I'm so confused. It says to use synthetic substitution which I thought meant to substitute x for 3, but when I turned in the assignment, I was supposed to use synthetic division. I just don't understand how to get the answer.

Answer 1

2157

Explanation:

The given function is
`f(x)=5x^5+10x^4+3x^3+8x^2-6x-3`

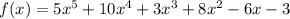
To find the remainder using synthetic division, we write the coefficient of this function in the top row as shown in the attachment.

The 5 in the bottom row is the result of adding 5 to 0 since there is nothing beneath 5.

We then multiply by the divisor(3) to obtain 15, which is written directly below 10 in the second row.

Add 10 and 15 to obtain 25.

Repeat the same process until you obtain the last number in the last row which is 2157, and this is your remainder.