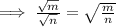Question

What are the rules for adding, subtracting, multiplying, and dividing radicals?

Answer 1

√m + √m = √2m ( CANNOT ADD √m + √n)

√m - √m = 0 ( CANNOT SUBTRACT √m + √n)

√m x √n = √mn

`\implies (√(m))/(√(n)) =\sqrt{ (m)/(n)}`

Explanation:

A number m which if in the ROOT form is called a RADICAL.

Example : √2, ∛8, √6 .. etc.

Here, the number Inside the root is called RADICAND.

Also, the number Outside the root is called INDEX.

ADDITION OF RADICALS:

The number that is under the radical must be the same for each radical in order to add two radicals.

⇒ We CAN add √3 and √3 but CANNOT add √3 and √5.

SUBTRACTING OF RADICALS:

The number that is under the radical must be the same for each radical in order to subtract two radicals.

⇒ We CAN Subtract √3 from √3 but CANNOT subtract √3 from √5.

MULTIPLICATION OF RADICALS:

To multiply any two radicals, simply MULTIPLY The NUMBERS inside the radical.

⇒√m x √n = √mn

DIVISION OF RADICALS:

To divide any two radicals, simply DIVIDE The NUMBERS inside the radical.

`\implies (√(m))/(√(n)) =\sqrt{ (m)/(n)}`