Answer: a) 0.996, b) 0.253, c) 0.02.
Explanation:
Since we have given that
Probability of success = Probability of the math majors belong to ethnic minorities = 33% = 0.33 =p
Probability of failure = q = 1-0.33 = 0.67
Number of students selected = 7
a) No more than 5 belong to an ethnic minority
So, using "binomial distribution", we get that

b. Exactly three of them belong to an ethnic minority
So, it becomes,
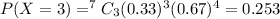
c. None of them belong to an ethnic minority
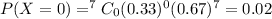
Hence, a) 0.996, b) 0.253, c) 0.02.