Final answer:
The equation of the line parallel to
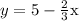 and passing through the point (-3,0) is
and passing through the point (-3,0) is
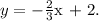
Step-by-step explanation:
To write the equation of a line that is parallel to the given line
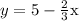 and passes through the point (-3,0), we first identify the slope of the given line. The slope (m) of the line
and passes through the point (-3,0), we first identify the slope of the given line. The slope (m) of the line
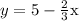 is \(-\frac{2}{3}\). Since parallel lines have the same slope, our new line will also have the slope of
is \(-\frac{2}{3}\). Since parallel lines have the same slope, our new line will also have the slope of
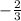 . Using the point-slope form of a line's equation, which is y - y1 = m(x - x1), where (x1, y1) is a point on the line and m is the slope, we substitute m with
. Using the point-slope form of a line's equation, which is y - y1 = m(x - x1), where (x1, y1) is a point on the line and m is the slope, we substitute m with
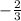 and
and
 with (-3,0):
with (-3,0):
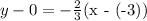
Simplifying, we get:

This is the equation of the line parallel to
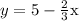 passing through the point (-3,0).
passing through the point (-3,0).