Question

What two fractions multiply to equal -1 and add to equal 1/4

Answer 1

The two such fractions are: 113/100 and -22/25.

Explanation:

Let us assume two such fractions are P and Q.

⇒ P x Q = -1

and P + Q = 1/4

Now, to solve for the values of P and Q:

P + Q = 1/4 ⇔ Q = 1/4 - P

Substitute this value of Q in P x Q = -1, we get:

P x Q = -1

`P * ((1)/(4) - P ) = -1\\\implies(P)/(4) -P^2 = -1\\\implies P - 4P^2 = -4\\\implies 4P^2 -P -4 = 0`

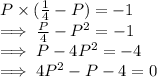
Now, solving this with the help of QUADRATIC Formula:

`x = (-b\pm√(b^2-4ac))/(2a)`

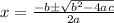
We get: P = 1.13 or P = -0.8825

Now, if P = 1.13, Q = -1/1.13 = -0.88 = -88/100 = -22/25

If P = -0.88, then Q = 1/0.88 = 1.13 = 113/100

Hence, The two such fractions are: 113/100 and -22/25.