Question

We collected a sample of the prices of new homes. The mean of our sample is $155,000, with a standard deviation of $15,000. Calculate the z-scores of each of the given prices. Determine if the they are usual or unusual. Round up each z-score to two decimals. (a) $200, 000 (b) $55,000 (c) $175,000 (d) $122,000

Answer 1

(a) $200, 000, z-score= 3 and it is unusual.

(b) $55,000, z-score= -6.67 and it is unusual.

(c) $175,000, z-score= 1.33 and it is usual.

(d) $122,000, z-score= -2.2 and it is unusual

Step-by-step explanation:

Given: Mean of sample= $155000

Standard deviation= $15000.

Now, calculating z-score of each given prices.

z-score=
`(x-mean)/(standard\ deviation)`

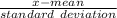
(a) Price= $200000

`z-score = (200000-155000)/(15000)`

⇒
`z-score= (\$45000)/(\$ 15000) = 3`

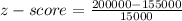
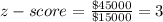
It is unusual as score is very high.

b) $ 55000

`z-score = (55000-155000)/(15000)`

⇒
`z-score = (-100000)/(15000)`

∴
`z-score= -6.67`

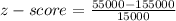
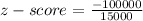
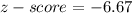
It is unusual again as score it very low.

c) $ 175000

`z-score = (175000-155000)/(15000)`

⇒
`z-score = (20000)/(15000)= 1.33`

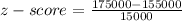
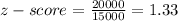
It is usual as score is in the top 0.30

d) $122000

`z-score = (122000-155000)/(15000)`

⇒
`z-score = (33000)/(15000)`

∴
`z-score= -2.2`

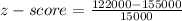
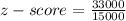
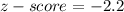
It is unusual as score is too low