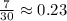Question

9. On a game show, there are 16 questions: 8 easy, 5 medium-hard, and 3 hard. If contestants are given questions

randomly, what is the probability that the first two contestants will get easy questions?

Answer 1

`(7)/(30)\approx0.23`

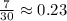
Explanation:

Given:

Number of questions (N) = 16

Number of easy questions (E) = 8

Number of medium-hard questions (M) = 5

Number of hard questions (H) = 3

Now, the probability of getting the first question as easy question is given as:

`P(E1)=\frac{\textrm{Number of easy questions}}{\textrm{Total questions}}\\\\P(E1)=(E)/(N)=(8)/(16)=0.5`

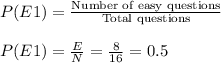
Now, probability of getting the second question as easy question is given as:

`P(E2)=\frac{\textrm{Number of easy questions left}}{\textrm{Total questions left}}\\\\P(E2)=(E-1)/(N-1)=(7)/(15)`

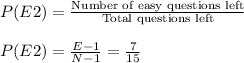
Now, probability that the first two contestants will get easy questions is given by the product of
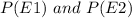`P(E1)\ and\ P(E2)`. So,

`P(2\ easy\ questions)=P(E1)* P(E2)\\\\P(2\ easy\ questions)=(1)/(2)* (7)/(15)\\\\P(2\ easy\ questions)=(7)/(30)\approx 0.23`

Therefore, the probability that the first two contestants will get easy questions is
`(7)/(30)\approx0.23`