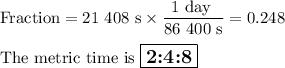Question

If 1 day was 10 metric hours, 1 metric hour was 10 metric minutes, and 1 metric minute was 10 metric seconds, what time would it really be if your metric clock reads 8:9:2? Similarly, convert 5:56:48 A.M. to metric time. You may assume that each new day starts at midnight.

Answer 1

`\large \boxed{\text{A. 21:24:29; B. 2:4:8}}`

Explanation:

A. From metric time to normal

In normal time,

1 min = 60 s

1 h = 60 min = 3600 s

1 day = 24 h = 1440 min = 86 400 s

A time of 8:9:2 corresponds to 0.892 day.

0.892 day = 0.892 × 86 400 s = 77 069 s

1. Seconds to hours

`\text{77 069 s} * \frac{\text{1 h}}{\text{3600 s}} = \text{21.408 h} = \text{21 h + 1469 s}`

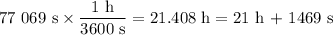
2. Seconds to minutes

`\text{1469 s} * \frac{\text{1 min}}{\text{60 s}} = \text{24.4833 min} = \text{24 min + 29 s}`

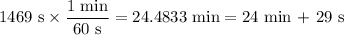
So, 0.892 day = 21 h + 24 min + 29 s

In international notation, the time is 21:24:29 (about 9:24 p.m.).

B. From normal time to metric

In metric time,

1 h = 0.1 day

1 min = 0.1 h = 0.01 day

1 s = 0.1 min = 0.01 h = 0.001 day

So, the hours, minutes and seconds are tenths, hundredths, and thousandths of a day.

1. Convert the time to seconds

5:56:48 = (5 × 3600 + 56 × 60 + 48) s = (18 000 + 3360 + 48) s = 21 408 s

2. Calculate the fraction of a day

`\text{Fraction} = \text{21 408 s} * \frac{\text{1 day }}{\text{86 400 s}} = \textmb{0.248}\\\\\text{The metric time is $\large \boxed{\textbf{2:4:8}}$}`