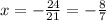Question

Which value of x satisfies both - 9x + 4y = 8 and -3x-y= 4 given the same value of y?

ܝܙܢ ܢܩ ܣܢ ܩ ܬ ܐܲ

Answer 1

`x=-(8)/(7)`

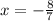
Explanation:

We want to find the value of x that satisfies both

- 9x + 4y = 8

and

-3x-y= 4

given the same value of y.

This is the x-value of the point of intersection of the graph of the two lines.

We make y the subject of the bottom equation to get:

y=-3x-4

We substitute y=-3x-4 into the top equation to get:

-9x+4(-3x-4)=8

Expand to get:

-9x-12x-16=8

Group like terms

`-9x-12x=8+16`

-21x=24

`x=-(24)/(21)=-(8)/(7)`