Question

write slope intercept form of equation for the line passing through the given points. and equation (-2,8) and (1,-3)

Answer 1

`\bf (\stackrel{x_1}{-2}~,~\stackrel{y_1}{8})\qquad (\stackrel{x_2}{1}~,~\stackrel{y_2}{-3}) ~\hfill \stackrel{slope}{m}\implies \cfrac{\stackrel{rise} {\stackrel{y_2}{-3}-\stackrel{y1}{8}}}{\underset{run} {\underset{x_2}{1}-\underset{x_1}{(-2)}}}\implies \cfrac{-11}{1+2}\implies -\cfrac{11}{3}`

`\bf \begin{array}c \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{8}=\stackrel{m}{-\cfrac{11}{3}}[x-\stackrel{x_1}{(-2)}]\implies y-8=-\cfrac{11}{3}(x+2) \\\\\\ y-8=-\cfrac{11}{3}x-\cfrac{22}{3}\implies y=-\cfrac{11}{3}x-\cfrac{22}{3}+8\implies y=-\cfrac{11}{3}x+\cfrac{2}{3}`

Answer 2

`y = -(11)/(3)x + (2)/(3)`

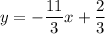
Explanation:

The slope-intercept equation for a straight line is

y = mx + b

where m is the slope of the line and b is the y-intercept.

The line passes through the points (-2, 8) and (1, -3)

(a) Calculate the slope of the line

`\begin{array}{rcl}m & = & (y_(2) - y_(1))/(x_(2) - x_(1))\\\\ & = & (-3 - 8)/(1 - (-2))\\\\& = & (-11)/(1 + 2)\\\\& = & \mathbf{-(11)/(3)}\\\\\end{array}`

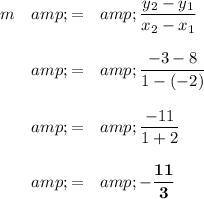
(b) Find the y-intercept

Insert the coordinates of one of the points into the equation

`\begin{array}{rcl}y & = & mx + b\\-3 & = & -(11)/(3)*1 + b\\ \\b & = & -3 + (11)/(3)\\\\& = & -(9)/(3)+ (11 )/(3)\\\\& = &\mathbf{(2)/(3)}\\\end{array}`

(c) Write the equation for the line

`\mathbf{y} = \mathbf{-(11)/(3)x + (2)/(3)}`

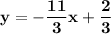
The diagram below shows your graph passing through the two points with a slope of -11/3 and a y-intercept of ⅔.