Answer:
5,230kgm^{-3}
Step-by-step explanation:
In physics, there are what we call prefix and SI units. Prefix are letters that actually denote a numerical value and this are most times appended before the SI unit of a physical quantity and SI unit is just a convention appended in the magnitude of a physical quantity so that this magnitude can be imagined. I will give quick examples, consider this magnitude of length:
200cm - it is pronounced 200 centi meter. In this case, the centi is actually a prefix denoting the value (
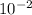 ) and the meter is the SI unit for length. So converting 200cm -> m is as simple as saying
) and the meter is the SI unit for length. So converting 200cm -> m is as simple as saying

So in this question, we want to convert
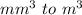
Now the prefix here is milli which is (
 ) and the SI unit is meter
) and the SI unit is meter
=>
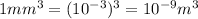
density =
