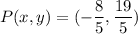Question

What are the coordinates of the point on the directed line segment from (-2, 9)(−2,9) to (-1, -4)(−1,−4) that partitions the segment into a ratio of 2 to 3?

Answer 1

Therefore the coordinates of the point on the directed line segment from (-2, 9) to (-1, -4) that partitions the segment into a ratio of 2 to 3 is

`P(x,y)=(-(8)/(5),(19)/(5))`

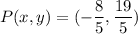
Explanation:

Given:

Let point P divides Segment AB in the ratio 2 : 3

point A( x₁ , y₁) ≡ ( -2 , 9 )

point B( x₂ , y₂) ≡ ( -1 , -4 )

m : n = 2 : 3

To Find:

P( x, y ) = ?

Solution:

Ia a Point P divides Segment AB internally in the ratio m : n, then the Coordinates of Point P is given by Section Formula as

`x=((mx_(2) +nx_(1)) )/((m+n))\\ \\and\\\\y=((my_(2) +ny_(1)) )/((m+n))\\\\`

Substituting the values we get

`x=((2(-1) +3(-2)))/((2+3))\\ \\and\\\\y=((2(-4) +3(9)) )/((2+3))\\\\`

`x=(-8)/(5)\\ \\and\\\\y=(19)/(5)\\\\`

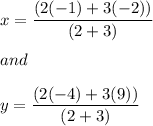
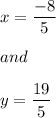
Therefore the coordinates of the point on the directed line segment from (-2, 9) to (-1, -4) that partitions the segment into a ratio of 2 to 3 is

`P(x,y)=(-(8)/(5),(19)/(5))`