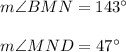Question

Two parallel lines are cut by a transversal and two of the same sides interior angle formed have measures of (4x+3)and(x+2)

A. how are the angles related?

B.write and solve an equation to find the value of x.

C.what are two angle measures?​

Answer 1

Therefore,

A. ∠BMN + ∠MND = 180°

B . x = 35

C.
`m\angle BMN = 143\°\\\\m\angle MND = 47\°`

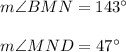
Explanation:

Given:

Consider the Figure below such that

AB || CD

PQ as transversal

m∠BMN = (4x+3) and

m∠MND = (x+2)

To Find:

1. Relation between interior angles

2. x = ?

3. m∠BMN = ? and m∠MND = ?

Solution:

Same Side Interior Postulate:

The same-side interior angle theorem states that "when two lines that are parallel are intersected by a transversal line, the same-side interior angles that are formed are supplementary, or add up to 180 degrees".

As, AB || CD

PQ as transversal

∠BMN and ∠MND are Same Side Interior angles.

∴ ∠BMN + ∠MND = 180° ......Relationship between the angles.

Substituting the values we get

`(4x+3)+(x+2)=180\\5x+5=180\\5x=175\\\\x=(175)/(5)=35`

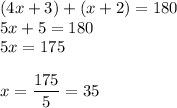
Substitute 'x' in ∠BMN and ∠MND we get

`m\angle BMN = 4* 35 +3=143\°\\\\m\angle MND = 45+2=47\°`

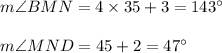
Therefore,

A. ∠BMN + ∠MND = 180°

B . x = 35

C.
`m\angle BMN = 143\°\\\\m\angle MND = 47\°`