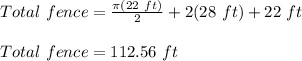Answer:
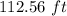
Explanation:
Observe the picture attached.
The circumference of a circlecan be found with the following formula:

Where "d" is the diameter of the circle.
The perimeter of a rectangle can be found by adding its sides.
Notice that in this case:
1. You need half the circumference.
2. You must not include one of its sides that measures 22 feet,
Then, with this information, you can identify that the the amount of fence (in feet) that the gardener requires to build the fence around the rose garden, is given by:
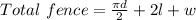
Where "l" is the lenght of the rectangle and "w" is the width.
Knowing that:
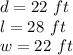
You can substitute values and evaluate.
The result is: