A heat energy equal to 6300 J is required to heat 10.75 g of ice to steam.
Explanation:
Given:
Amount of transferred energy = Q =?
Mass of ice (water) = m = 10.75 g
Initial temperature of ice =
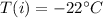
Final temperature of steam =
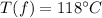
Formula for Heat capacity is given by
Q = m×c×Δt ........................................(1)
where:
Q = Heat capacity of the substance (in J)
m=mass of the substance being heated in grams(g)
c = the specific heat of the substance in J/(g.°C)
Δt = Change in temperature (in °C)
Δt = (Final temperature - Initial temperature) =
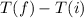
Specific heat of water is c = 4.186 J /g. °C
Substituting these in equation (1), we get
Q = m×c×Δt =
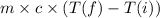

Required heat energy is equal to 6299.93 Joules ≅ 6300 Joules.