 is the factored form of
is the factored form of
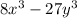
Solution:
We have to factor the given expression
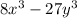
We can use the algebraic identity to factor the given expression
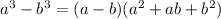
Lets make the given expression in form of
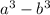
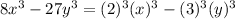

Now apply the algebraic property in above expression
Here, a = 2x and b = 3y
Therefore,
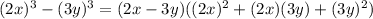

Thus the given expression is factored out