Question

Find the quotient of these complex numbers (4-6i)/(2-5i)

Answer 1

`(38)/(29) + (8)/(29)i`

Explanation:

We have to find the quotient of the division of complex numbers
`((4 - 6i))/((2 - 5i))`.

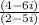Now, we have to get the expression in simplified form.

`((4 - 6i))/((2 - 5i))`

=
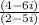
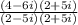`((4 - 6i)(2 + 5i))/((2 - 5i)(2 + 5i))` {Rationalizing the denominator}

=
`(4(2 + 5i) - 6i(2 + 5i))/(2^(2) - (5i)^(2))`

=
`(8 + 20i - 12i - 30(i)^(2))/(4 + 25)`

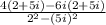
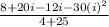
{Since, i = √(- 1) i.e. square root of negative one, hence, i² = - 1}

=
`(8 + 30 + 8i)/(29)`

=
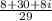
`(38)/(29) + (8)/(29)i` (Answer)