Question

The front of an A-frame cabin in a national park is the shape of a triangle with an area of 564 ft.² if the height is 1 feet less than twice the base find the base and the height of the front of the cabin

Answer 1

Base = 24 ft; height = 47 ft

Explanation:

The formula for the area of a triangle is

A = ½bh

Let x = the base. Then

2x - 1 = the height

`\begin{array}{ccc}A & = & (1)/(2)bh\\\\564 & = & (1)/(2)x(2x - 1)\\ \\1128 & = & x(2x - 1)\\& = & 2x^(2) - x\\2x^(2) - x - 1128 & = & 0\\(2x + 47)(x - 24) & = & 0\\2x + 47 = 0 &\qquad & x - 24 = 0\\2x = -47 & \qquad & x = \mathbf{24}\\x = -23.5 & \qquad & \\\end{array}`

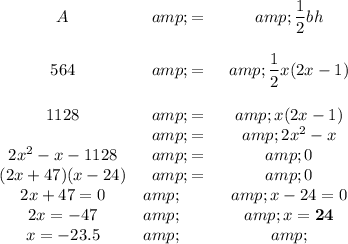
We reject the negative value, so x = 24 ft

Base = x = 24 ft

Height = 2x - 1 = 2(24)-1 = 48 - 1 = 47 ft

Check:

564 = ½ × 24 × 47

564 = 564

OK