Answer:
See explanation
Explanation:
The given function is
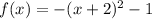
You could observe that:
 when you compare to
when you compare to
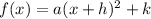 .
.
This should tell you that the graph should open downwards.
Let us substitute
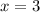 , to get:
, to get:
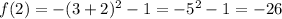
Also when x=4,
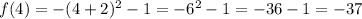
All the results are negative so the graph should appear below the x-axis
This shows that AJ made a mistake in the graph.