Answer:
y=32, x=96
Explanation:
The complete question in the attached figure
we know that
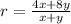 ----> given problem
----> given problem
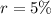 ----> given problem
----> given problem
substitute the value of r in the expression
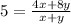
Simplify the expression
Multiply both sides by (x+y)
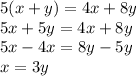
So
In the table, just look for two values, one of which is three times the other
Verify each number in the table
1) For y=21 ----> x=3(21)=63
The value of 63 is not in the table
2) For y=32 ----> x=3(32)=96
The value of 96 is in the table
3) For y=51 ----> x=3(51)=153
The value of 153 is not in the table
4) For y=64 ----> x=3(64)=192
The value of 192 is not in the table
5) For y=81 ----> x=3(81)=243
The value of 243 is not in the table
6) For y=96 ----> x=3(96)=288
The value of 288 is not in the table
therefore
y=32, x=96