Question

Two tiny, spherical water drops, with identical charges of -4.29 Ã 10-16 C, have a center-to-center separation of 0.845 cm. A) What is the magnitude of the electrostatic force acting between them? B) How many excess electrons are on each drop, giving it its charge imbalance?

Answer 1

Electrostatic force,
`F=2.31* 10^(-17)\ N`

Number of electrons, n = 2681 electrons

Step-by-step explanation:

Given that,

Charges,
`q_=q_2=-4.29* 10^(-16)\ C`

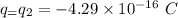
Separation between charges,
`r=0.845\ cm=0.845* 10^(-2)\ m`

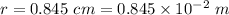
(a) Let F is the magnitude of the electrostatic force acting between them. The electric force between charges is given by :

`F=(kq^2)/(r^2)`

`F=(9* 10^9* (4.29* 10^(-16))^2)/((0.845* 10^(-2))^2)`

`F=2.31* 10^(-17)\ N`

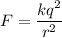
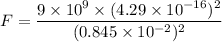
(b) Let n be the excess electrons on each drop, giving it its charge imbalance. It can be calculated using quantization of electric charge as :

`q=ne`

`n=(q)/(e)`

`n=(4.29* 10^(-16))/(1.6* 10^(-19))`

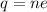
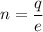
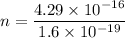
n = 2681.25 electrons

or

n = 2681 electrons

So, the number of excess electrons on each drop is 2681 electrons. Hence, this is the required solution.