Question

For any degenerate pair of states in 2D infinite potential well, what is the maximum number of electrons allowed?

a) 1

b) 2

c) 3

d) 4

e) 5

f) 6

Answer 1

The correct answer is b, two electrons

Step-by-step explanation:

The energy in a well of infinite power in one dimension is

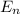`E_(n)` = (h² / 8mL²) n²

In the case of a well with two dimensions the energy is given by the same relationship in each dimension

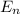`E_(n)` = (h² / 8 m L²) (n₁² + n₂²) = E₀ (n₁² + n₂²)

For simplicity, suppose that the length of the well in the home dimension is the same

This energy is degenerated because several combinations of quantum numbers give the same energy value.

Let's calculate the quantum energy and number for several states

n₁ n₂
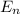`E_(n)` / E₀

1 1 2 not degenerated

2 1 5

1 2 5 this two level is degenerated,

2 2 8 not degenerated

3 1 10

1 3 10 these two are degenerated

3 2 13

2 3 13 twon level degenerated

3 3 18 not degenerate

From this table we see that the states with equal quantum numbers are not degenerated and the states with different quantum numbers are degenerated into pairs, as in each state an electron fits in the two states two electrons fit

The correct answer is b