Jane needs to run 22 laps to cover 4 miles
Solution:
From given question,
Jane is running on a circular track with a radius of 50 yards
Radius = 50 yards
The total distance covered in 1 lap is equal to circumference of circular track
The circumference of circle is given as:
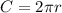
Where, "C" is the circumference and "r" is the radius
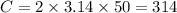
Thus one lap is equal to 314 yards
Convert yards to miles
Given that,
1 mile = 1760 yards
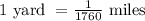
Therefore, 314 yards is equal to,

What is the least number of complete laps she must run to cover a distance of at least 4 miles?
In 1 lap, she covers 0.1784 miles
Let "x" be the number of laps needed to cover 4 miles
Therefore,
1 lap = 0.1784 miles
x lap = 4 miles
This forms a proportion and we can solve the sum by cross multiplying
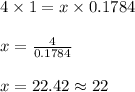
Thus she needs to run 22 laps to cover 4 miles