Question

A rocket is fired vertically upward. At the instant it reaches some altitude with a speed of 100 m/s. It explodes into three fragments having equal mass. One fragment moves upward with a speed of 150 m/s following the explosion. The second fragment has a speed of 150 m/s and is moving east right after the explosion. What is the velocity of the third fragment immediately after the explosion?

Answer 1

The magnitude of the velocity of the third fragment is 212.13 m/s.

Step-by-step explanation:

Given that,

Speed = 100 m/s

Vertical speed of first fragment = 150 m/s

Vertical speed of second fragment = 150 m/s

We need to calculate the vertical speed of third fragment

Using conservation of momentum

`P_(iy)=P_(fy)`

`mv=(m)/(3){v_(1)}+(m)/(3)v_(2)+(m)/(3)v_(3)`

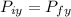
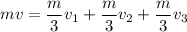
Put the value into the formula

`m*100=(m)/(3)*150+(m)/(3)*0+(m)/(3)* v_(y)`

`(1)/(3)v_(y)=100-50`

`v_(y)=150\ m/s`

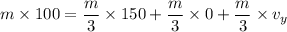
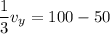
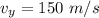
We need to calculate the horizontal speed of third fragment

Using conservation of momentum

`P_(iy)=P_(fy)`

`mv=(m)/(3){v_(1)}+(m)/(3)v_(2)+(m)/(3)v_(3)`

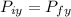
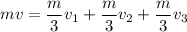
Put the value into the formula

`m*0=(m)/(3)*0+(m)/(3)*150+(m)/(3)v_(x)`

`(1)/(3)v_(x)=-50`

`v_(x)=-150\ m/s`

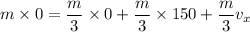
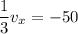
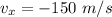
We need to calculate the magnitude of the velocity of the third fragment

`v=\sqrt{v_(x)^2+v_(y)^2}`

Put the value into the formula

`v=√((-150)^2+(150)^2)`

`v=212.13\ m/s`

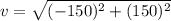
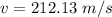
Hence, The magnitude of the velocity of the third fragment is 212.13 m/s.