Question

Carl drew a rectangle with a width of 8 in. and a length of 32 in. Which rectangles are NOT similar to Carl's rectangle? Select ALL that apply. Question 1 options: a rectangle with a width of 10 cm and a length of 44 cm a rectangle with a width of 2.5 in and a length of 10 in a rectangle with a width of 23 cm and a length of 92 cm a rectangle with a width of 9 mm and a length of 45 mm

Answer 1

The rectangles that are not similar to Carl's rectangle are:

A rectangle with width of 10 cm and length of 44 cm

A rectangle with width of 9 mm and length of 45 mm

Solution:

Carl drew a rectangle with a width of 8 in. and a length of 32 inches

Length of the rectangle = 32 inches

Width of the rectangle = 8 inches

Find the ratio of length by width

`(length)/(width) = (32)/(8) = (1)/(4)`

`(length)/(width) = (4)/(1) -------------- equation 1`

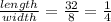
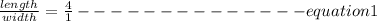
So the ratio of length to width is 1 : 4

When ratio of the dimension of corresponding rectangles are equal then the two rectangles are said to be similar.

Check the given options and find which has the similar ratio of length and width

1) A rectangle with width of 10 cm and length of 44 cm

1 cm = 0.393701 inches

width = 10 cm = 3.93701 inches

length = 44 cm = 17.3228 inches

`(length)/(width) = (17.3228)/(3.93701)`

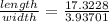
This is not equal to eqn 1

Thus this rectangle is not similar to Carl's rectangle

2) A rectangle with width of 2.5 inch and length of 10 inch

`(length)/(width) = (10)/(2.5) = (100)/(25) = (4)/(1)\\\\(length)/(width) = (4)/(1)`

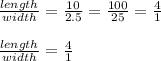
This is equal to eqn 1

This rectangle is similar to Carl's rectangle

3) A rectangle with width of 23 cm and length of 92 cm

1 cm = 0.393701 inches

width = 23 cm = 9.05512 inches

length = 92 cm = 36.2205 inches

`(length)/(width) = (36.2205)/(9.05512) = (4)/(1)`

`(length)/(width) = (4)/(1)`

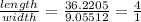
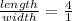
This is equal to eqn 1

This rectangle is similar to Carl's rectangle

4) A rectangle with width of 9 mm and length of 45 mm

1 mm = 0.0393701 inches

length = 45 mm = 1.77165 inches

width = 9 mm = 0.354331 inches

`(length)/(width) = (1.77165)/(0.354331) = (5)/(1)`

This is not equal to eqn 1

Thus this rectangle is not similar to Carl's rectangle