Question

Three quarters of the boats in the marina are white. 4/7 of the remaining boats are blue and the rest are red. If there are 9 red boats how many boats are in the marina?

Answer 1

84 boats are there in the marina.

Explanation:

Given:

Three quarters of the boats are white.

4/7 of the remaining boats are blue and rest are red.

Number of red boats (r) = 9

Total number of boats = ?

Let the total number of boats be 'x'.

As per question, three quarters are white. So,

Number of white boats (w) =
`(3)/(4)* x=(3x)/(4)`

Now, boats remaining are given as:

Remaining boats = Total boats - White boats

Remaining boats =
`x-(3x)/(4)=(4x)/(4)-(3x)/(4)=(4x-3x)/(4)=(x)/(4)`

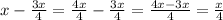
As per question, 4/7 of the remaining boats are blue.

Number of blue boats (b) =
`(4)/(7)* (x)/(4)=(x)/(7)`

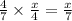
Now, the rest are red. So,

Number of red boats (r) = Total number - ( White boats + Blue boats )

`r=x-(w+b)`

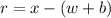
Plug in 9 for 'r',
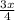`(3x)/(4)` for 'w' and
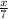`(x)/(7)` for 'b'. Solve for 'x'. This gives,

`9=x-((3x)/(4)+(x)/(7))\\\\9=x-((3x* 7)/(4* 7)+(x* 4)/(7* 4))\\\\9=x-((21x)/(28)+(4x)/(28))\\\\9=x-(21x+4x)/(28)\\\\9=(28x)/(28)-(25x)/(28)\\\\9=(28x-25x)/(28)\\\\9* 28=3x\\\\x=(9* 28)/(3)=3* 28=84`

Therefore, the total number of boats in the marina are 84.